Research Bio
Applications of computational schemes for partial differential equations to such areas as semiconductor manufacturing, two and multi-phase fluid flows, industrial processes, including microjetting and efficient combustion, optimization in structural design, image segmentation in medical imaging and inverse problems, wave propapagation. Inverse problems and data extraction from experiment, especially those from synchrotron science and microscopy.
Research Expertise and Interest
mathematics, applied mathematics, partial differential equations, computational physics, level set Methods, computational fluid mechanics and materials sciences, fast marching methods
In the News
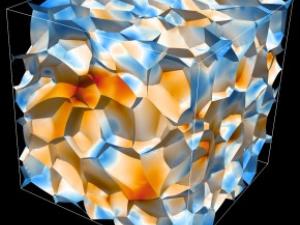
Heady mathematics: Describing popping bubbles in a foam
Two University of California, Berkeley, researchers have now described mathematically the successive stages in the complex evolution and disappearance of foamy bubbles, a feat that could help in modeling industrial processes in which liquids mix or in the formation of solid foams such as those used to cushion bicycle helmets.
Three faculty members named to National Academy of Sciences
In recognition of their excellence in original scientific research, three UC Berkeley faculty members have been elected members of the National Academy of Sciences (NAS), one of the highest honors given to a scientist or engineer in the United States.
Berkeley Lab mathematicians win Cozzarelli Prize
Berkeley Lab mathematicians James Sethian and Robert Saye have won the 2011 Cozzarelli Prize for the best scientific paper in the category of Engineering and Applied Sciences.
Mathematicians awarded prizes for work on fluid mechanics, interface dynamics
Alexandre Chorin and James Sethian, two UC Berkeley and LBNL mathematicians, will receive awards from the world's largest applied mathematics society for their career and pioneer contributions to the field.